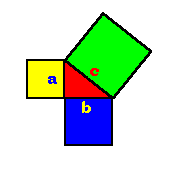The Pythagorean theorem was first known in ancient Babylon and Egypt (beginning about 1900 B.C.). The relationship was shown on a 4000 year old Babylonian tablet now known as Plimpton 322. However, the relationship was not widely publicized until Pythagoras stated it explicitly.

Pythagoras lived during the 6th century B.C. on the island of Samos in the Aegean Sea. He also lived in Egypt, Babylon and southern Italy. Pythagoras was a teacher and a philosopher.

Pythagoras found out that for a right angle triangle (with one of the angles being 90^o), the square of the hypotenuse is equal to the sum of the squares of the other two sides: a²+b²=c².

In other words, the green square's area (with area c²) equals the sum of two others. It is exactly the yellow square's area (a²) plus the blue square's (b²).

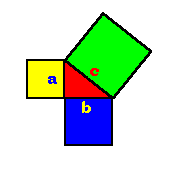
Now take a look at the tile pattern.

[The proof of Pythagorean Theorem is in the following figure.]

Count the triangles within the squares. The number of green triangles equals the total of those that are yellow and blue!

Do You Know ...

Archeologists use the Pythagorean in field excavations. When they start a dig, they place a rectangular grid over the site surface. To lay out an accurate grid system, archeologists use the Theorem, X ²+Y²=Z². After deciding how long the baselines (X- and Y- axis) should be, the proper length of the diagonal is calculated using the Pythagorean theorem to make sure the quadrant is a rectangle and not a lopsided parallelogram. Corner stakes will then be placed to mark the accurate location of the site.

An overlaying rectangular grid uses a mathematical idea called Cartesian coordinate system.