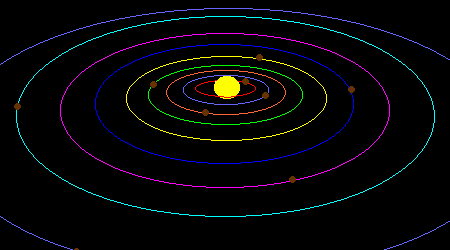
For many centuries people sought knowledge about motion of objects in the night sky as ways to understand our universe. One man found that the planets revolve around the sun not in circles, but in a general kind of oval. The name of that shape is ellipse.

The phenomonen was discovered by Johan Kepler, a German astronomer who lived from 1571 to 1630 A.D. He established 3 laws describing how planets move.

All the shapes below in the figures are ellipses. The outer ones are familiar to us as circles. Circles are ellipses: they are just a special kind.

Ellipses with the same vertices

Ellipses with the same focal points

In an ellipse, the total distance between any point on the outline and the two focal points is a constant.

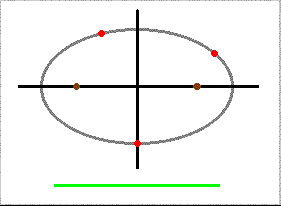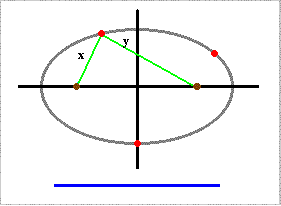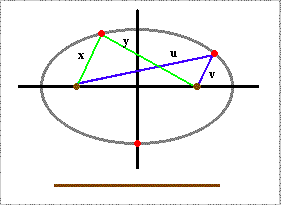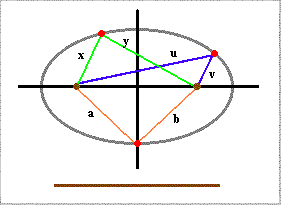
x + y = u + v = a + b = constant
(the total lengths are the same)

In circles the focal points lie on top of one another, so we only have one, the center. The center-to-boundary distance in a circle is the same for every point on the periphery.